OVERHEAD ALLOCATION

Models for Developing the Overhead Allocation Factor

Let me reiterate - the business management purpose of using a model to derive an Overhead Allocation Factor is to get to a viable, reasonably accurate number, or factor, to use in doing the next estimate.

I have included some Allocation Factor models below. The models are meant to derive a factor which will be applied to your estimates to account for overhead expenses accrued during the performance of a job.

There is no reason to expect that the Overhead Allocation Factor will stay static during a year. Unless you are going to use the Overhead Allocation information to adjust your Overhead Factor used in your estimating process, it is probably not worth the effort to break the overhead expenses into the various job accounts. The overhead expenses will show up in the income statement anyway, and re-allocating those expenses on a periodic basis is simply an accounting exercise if you do not actively use the results to adjust your Overhead Factor.

The next two pages in this series deals with how to actually account for the Overhead Expenses. We have already set the stage for that effort by discussing the addition of two accounts to your chart of accounts. On the next two pages, we will look at two smoothing methods for periodically updating the actual Overhead Expenses and the Overhead Allocation Factor to keep your estimated overhead expenses per estimate current.

Here are some Allocation Factor Models I have seen in use

Fixed Percent of Sales

A standard percentage (usually 10%) is used as an estimate for overhead expenses on the proposal. This percentage is multiplied by the Estimate Total to calculate Overhead Expense line item for the job.

Total Job Expenses X (1+Overhead Allocation Factor) = Total Job Costs

Total Job Costs X (1 + Profit Factor) = Proposal $

The advantage of this model is that it is easy to calculate (because you just make it up) and easy to apply (because you never adapt it to your business reality).

The disadvantage is that it does not reflect your real overhead costs, except by accident. Like the stopped watch that is right twice a day, this model may be close to reflecting actual costs, but if it is, it is almost an accident.

Historic Percent of Cost of Goods Sold

In this model, last year's results are used to develop an Overhead Factor which can be applied to new estimates. The formula is:

Overhead Expenses/Cost of Goods Sold = Overhead Factor%

and returns a percentage which is used as the Overhead Factor for estimating.

The Direct Costs of a project are calculated and then that aggregate number is multiplied by the Overhead Factor to determine the Overhead Load:

(Direct Labor, Direct Materials, Other Direct Costs) X Overhead Factor %= Overhead $

which is added to the Direct Costs and the Profit to determine the dollar value of the estimate:

(All Direct Costs + Overhead $) X (1 + Profit Factor) = Proposal $

The positive side of this model is that the Overhead Factor is developed from your own business experience. If the ratio was valid last year, it could be valid again this year.

Of course, any significant changes in business structure, or hiring, or your market, or your product will have major implications for your overhead costs. If you choose this model, you are forced to make arbitrary adjustments (increases or decreases) in the Overhead Factor to account for the changes you perceive, a process which decreases the efficacy of the model.

Baseline Dollars Per Job/Equal Distribution

This model is based on an assumption that all the projects done over the course of the year will require about the same overhead expenditures, so just calculate a number and assess that amount of overhead against every job. The formula looks like this:

Total Overhead Expenses last year/Total number of Jobs Last Year = Overhead Allocation per Job Last Year

and will return a number which can be applied to each job as the overhead charge for this year. Then,

(Total Direct Costs + Overhead Allocation Per Job Last Year) X (1+Profit Factor) = Proposal $

The fact that this model is based on historical numbers is both a pro and a con. At least there is some basis for the allocation amount, but, being historical, that number does not reflect current realities.

This model also assumes that all projects have the same overhead requirements. Unless you are building the same model over and over again, this assumption will be false.

If you use this method, you will theoretically win big jobs (for which you will have allocated too few dollars in Overhead Allocation) and lose small jobs (for which you added too many dollars in Overhead Allocation).

Don't use this allocation model.

Estimated Time to Build

This model is interesting in that it begins to recognize that there are elemental differences between projects, and it attempts to factor in those recognized differences.

The elemental difference with which this model deals is the time required to produce the income.

This model also assumes that fixed expenses, of which overhead expenses are substantially comprised, are repetitive in nature. That is, that rent is going to be the same each month, that management salaries will be the same each month, and so on.

The formula used is a bit more complex, and looks like this:

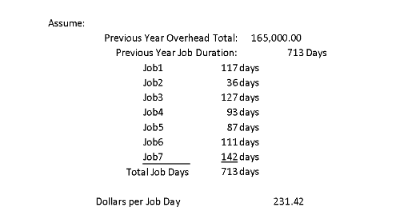
Last Year's Overhead Expenses/Last Year's Job Days = $ per Job Day

where Last Year's Job Days = (Days to complete Job1)+(Days to Complete Job2)+...(Days to Complete Job(n))

where "job day" is defined as a day within the period that a job started and that same job was completed

and is going to return a number representing the overhead dollars spent every job day.

For example, if a job was begun on March 3 and completed on June 27, that job would account for 116 Job Days. It is important to note that the year is not limited to 365 job days, as several jobs could be running on any one job day, and each would accrue one job day on that date.

Here's an example:

In the preparation of an estimate, you include an estimate as to the number of days the job will take. To find the overhead to be charged to the job being estimated:

Job Days x Dollars per Job Day = Overhead Charge

For instance, assume the job you are currently estimating will take 98 days to complete:

98 days x 231.48 per day = $22,680 Overhead charge

Then

(Total Direct Costs + $22,680) X (1 + Profit Factor) = Proposal $

This model still relies on historical data, in that it uses last year's overhead and last year's days per job. However, it does provide a more nuanced overhead estimate because it recognizes that some jobs require more time, and consequently more overhead expenditures, than others.

Use this model if you don't prepare a budget.

Time To Build - Using Budgeted Overhead

A further refinement of the "Estimated Time to Build" model would be to use this year's budgeted overhead expenses in place of last year's overhead expenses.

Again, remember that the purpose of these models is to develop an Overhead Factor which can be applied to estimates. We want to come up with a number which is meaningful and useful, in that it assigns a relatively accurate amount of overhead to an estimate to help us to recover indirect costs from the projects we sell.

Using the same base numbers as in the example above, but assuming that you expect to spend an additional $16,000 in overhead expenses this year, the dollars per job day would be computed as follows:

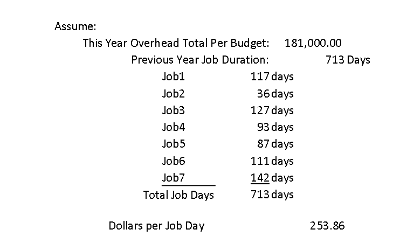
and would result in a different "Dollars per Job Day" assessment against estimated jobs.

Estimated Number of Job Days in Project X Calculated Dollars per Job Day = Overhead Allocation $

Then,

(Total of Direct Expenses + Overhead Allocation $) X (1 + Profit Factor) = Proposal $

Obviously, this model can only be used if a budget for the current year has been created. This process has the advantage of this year's overhead budget numbers being more current than last year's overhead expense numbers, and, if you have made any managerial changes which show up in the budget, those changes will be factored into the current budget numbers.

A Shortcut..

Once you have been tracking Job Days for a number of periods, you should be able to forecast with some degree of comfort the number of Job Days expected for the budget year, and simply divide the forecast Overhead Expenses by the forecast Job Days to return the "Per Job Day Overhead Allocation" for estimates.

Budgeted Overhead Expenses/Budgeted Job Days = Per Job Day Overhead Allocation

Then,

Per Job Day Overhead Allocation X Estimated Job Days for Project = Overhead Allocation $

And,

(Total Direct Costs + Overhead Allocation $) X (1 + Profit Factor) = Proposal $

How to Enter Overhead Allocations to Job Costing

When the time comes to allocate actual Overhead Expenses, there is a shortcoming which is inherent to any model. In months when you are very busy and in months when you are very slow, you will get whipsawed in your allocation amounts.

Assume, for instance, that you have Overhead expenses of $12,000/month. In a month when you have two houses going, your monthly overhead allocations would be $6,000 per house per month. In a month when you have six houses under construction, your monthly overhead allocations would be $2,000 per house per month. Neither of these extremes is accurate, and both will be troublesome if relied upon for estimating purposes.

What you need is a way to combine the high and low productivity months to arrive at a "smoothed" historical record upon which you may rely.

The next pages demonstrate two methods of actually allocating Overhead Expenses to jobs. Both employ smoothing techniques to adjust the periodic allocations for very busy months or very slow months. The first is the Overhead Allocation method based on Cost of Goods Sold (COGS) and smoothed for 12-month period. The second is the Overhead Allocation method based on Job Days, also smoothed for 12-month period.

Both also adjust the Overhead Allocation Factor for each period in an attempt to provide a better overhead allocation process for estimates. Simply adjust the Overhead Allocation Factor used by your estimators at the beginning of each month to reflect the smoothed number derived from the previous month's smoothed Factor calculation.

Return to ---->